8 Best Online Calculus Courses for 2023 | From Basic to Advanced

Calculus is a branch of mathematics focused on derivatives and integrals. Currently, we use calculus even in numerous fields such as engineering, physics, medicine, economics, and many more.

Undoubtedly, students who want to major in those fields need to take calculus courses before proceeding to more specialized courses. For instance, my major was financial economics in college. I had to take as many as three calculus courses to fulfill the requirement.

Though I am a math-lover, I admit that calculus is not easy to master, particularly if your instructor’s teaching skills are poor.

In my case, despite having attended the lectures, I had to spend hundreds of hours reading calculus books and working on thousands of practice problems. Fortunately, I got an A from that course.

I would say this strategy is effective in helping me master calculus. However, this strategy is exceptionally time-consuming.

High school and college students are already overwhelmed by the workload from other courses. You just cannot allocate that much time to learning calculus. Otherwise, your grades will be ruined.

Based on my observations, it would be better to enroll in online calculus courses taught by instructors with exceptional teaching skills. You will understand calculus concepts much faster and be able to score well on the exam.

This article will discuss the best online calculus courses that you can rely on. You can then select the course that suits your level and preferences and start learning right away.

Affiliate Disclosure: This article from Victory Tale contains affiliate links. We will receive a small commission if you purchase or subscribe to calculus courses through them.

Nonetheless, we always value integrity and prioritize our audience’s interests. You can then rest assured that we will present each course truthfully.

Things You Should Know

Prerequisites

Calculus is not for absolute beginners. To take a beginner-level calculus course, you should be familiar with the following:

• Algebra
• Trigonometry

However, you don’t need to excel in both. If you have taken a high-school course on them, that will suffice.

Advanced courses will have their own prerequisites. Please refer to the course section below for more information.

Criteria

Below are the criteria for the best online calculus courses.

You Still Need to Practice

Although online courses can help you understand calculus concepts in no time, you still need to practice by completing problem sets.

Unless you practice adequately, I can guarantee that you will have no chance to score well despite taking several online calculus courses.

Basic Calculus Courses

Courses under this headline will mostly cover a single variable calculus. You will learn about limits, differential calculus, and integral calculus or, in essence, Calculus I and II in college.

Note: Students who plan to take the AP Calculus Exam (AB/BC) can take the below courses to prepare for the exam.

1. Introduction to Calculus

This Coursera course from the University of Sydney is one of the best options for absolute beginners to learn calculus. Unlike other courses, this one includes precalculus and even algebra fundamentals in its curriculum.

Hence, you will learn everything from the beginning, which is excellent if you are not confident in your existing math skills.

Course Content

Below is a summary of topics you will learn from this 59-hour course.

• Precalculus – Algebraic Expressions, Equations and Inequalities, Coordinate Systems, Pythagoras’s Theorem, etc.
• Functions (Useful and Important Reportire) – Quadratic, Cubic, Polynomial, exponential/logarithmic, trigonometric functions, Composite Functions
• Introducing Differential Calculus – Slopes, Limits, Finding Derivatives, Leibniz Notation, Applications of Differentials
• Properties and applications of the derivative – Sign Diagrams, Minimum & Maximum, Concavity & Inflections, Curve Sketching, Chain/Product/Quotient Rules
• Introducing Integral Calculus – Slope of Tangent Lines, Areas Under Curves, Areas & Derivatives, The Fundamental Theorem of Calculus, Definite/Indefinite Integrals, Odd & Even Functions, The Logistic Function

In addition to well-made video lectures, this course will provide you with an excellent set of course materials, including course notes and reading references that you can always return to if you get stuck.

Best of all, this course also comes with 100+ practice problems that you can complete to sharpen your skills. With these resources, you may not need to buy an expensive calculus textbook at all.

You can audit this course for free. However, you can also opt for a full course to access graded assignments and a digital certificate by paying only $49 (one-time).

Pros & Cons

2. Become A Calculus Master Series by Krista King

Suppose you want less formal calculus training. In this case, I recommend taking this Udemy course from Krista King.

Krista is a veteran math tutor with exceptional teaching skills. She will guide you through all the concepts and provide excellent tips and tricks to help you score high on the calculus exam.

The series consists of three video courses, covering topics from limits to multivariable calculus. Thus, you can master all college-level calculus by completing the series.

Note: Udemy does not offer bundle pricing. You will need to buy each course separately.

2.1) Calculus I

This 12.5-hour course will cover Calculus I content, including the following topics:

• Limits & Continuity – Definitions, Combinations, Composites, Point/Jump/Infinite/Endpoint Discontinuities, Intermediate Value Theorem, Solving Limits, Squeeze Theorem
• Derivatives – Definitions, Rules, Chain Rule, Other Derivatives, Tangent and Normal Lines, Implicit Differentiation
• Applications of Derivatives – Optimization and Sketching Graphs, Linear Approximation, Related Rates, Applied Optimization, Derivative Theorems, Physics and Economics, Exponential Growth and Decay

Reviews: 4.7/5.0, Students: 60,700+

2.2) Calculus II

As its name suggests, this course will cover all Calculus II concepts and applications. The first part will delve into integration methods and other relevant theories as follows:

• Antiderivatives and Indefinite Integrals & Definite Integrals
• Riemann Sums, Other Approximation Methods, and Error Bounds
• Fundamental Theorem of Calculus and U-Substitution
• Integration by parts & Practical Fractions
• Trigonometric Integrals, Hyperbolic Integrals, Trigonometric Substitution, Improper Integrals, Reduction Formulas

The second part will discuss applications of integrals in several fields, including:

• Area Between Curves, Arc Length, Average Value
• Surface Area/Volume of Revolution
• Applications of Integrals in Geometry, Physics, Economics, and many more

The third part will discuss advanced integral concepts as follows:

• Parametric and Polar Curves
• Sequences and Partial Sums
• Geometric/Telescoping Series
• Basic Convergence, Comparison, Ratio and Root, Alternating Series Tests
• Power Series, Taylor Series, Maclaurin Series

The video content for this course is 30.5 hours long, far exceeding the former course. I like how the sequences and series sections are hugely informative since these concepts tend to confuse many learners.

Reviews: 4.8/5.0, Students: 38000+

2.3) Calculus III

This 24-hour course will go over advanced calculus concepts, particularly multivariable calculus and vector calculus. Below is what you will learn from this course:

• Three Dimensional Coordinate Systems
• Sketching Graphs and Level Curves
• Lines and Planes
• Partial Derivatives, Differentials, Chain Rule, Directional Derivatives
• Linear Approximation and Linearization, Gradient Vectors, Tangent Planes, and Normal Lines
• Optimization and Lagrange Multipliers
• Deep dive into multiple integrals (Double Integrals, Triple Integrals, Change of Variables, Applications, etc.)
• Vector Fundamentals (Dot/Cross Products, Vector Functions, Derivatives and Integrals, Arc Length and Curvature)
• Line Integrals, Stokes’ and Divergence Theorems

Reviews: 4.8/5.0, 29400+ students

Besides the video lectures, Krista provides detailed course notes and practice exercises in every course. The former is available for every calculus topic, while the latter is numbered as many as 600-800 per course.

In other words, if you buy all three courses, you will have 2000+ practice problems in your arsenal. Besides that, each course has a final exam. Hence, these helpful learning resources are more than sufficient for exam review.

The best thing about this series is that she explains everything in plain English. She will approach calculus concepts in a straightforward, step-by-step manner. Thus, you will grasp the knowledge far quicker than learning through college courses.

However, Krista will not prove most of the mathematical concepts. Instead, she will focus primarily on solving calculus problems. Thus, this series cannot replace your full-fledged calculus curriculum in college. You should then use the series as a prep course (to prepare for an upcoming final exam.)

Pros & Cons

3. XSeries Program in 18.01x Single Variable Calculus

This edX program is another excellent option to learn calculus online. Your instructors are faculty members of MIT, a world-renowned research university.

Course Content

This program consists of three following courses that you should take in order.

1. Calculus 1A Differentiation – The first course will drill deep into differentiation. You learn about its mathematical notation, physical meaning, and geometric interpretation. Later, you will learn to compute them in detail and grasp all of its significant use cases in specific fields.

2. Calculus 1B Integration – The second course will discuss everything related to integration. You will learn about its connection to derivatives and various methods to compute them.

Subsequently, you will learn to use integral calculus to illustrate real-world phenomena and complete several compelling tasks, such as finding a center of mass and a distance traveled by rocket.

3. Calculus 1C Coordinate Systems & Infinite Series – You will explore the significance of changing perspectives with coordinate systems and infinite series. Both of which are instrumental in making accurate computations.

MIT recommends spending 6-10 hours per week for ten months to complete the program. Unlike most other edX programs, this one is instructor-led. Thus, you cannot set your own study pace. You will then need to ensure that you have sufficient time to complete the assignments.

Auditing this program is free, but you will not be able to access graded assignments, which is necessary for learning math. I thus suggest subscribing to a verified track that costs $225 and provides full access to assignments and a digital certificate.

Pros & Cons

4. Brilliant’s Calculus Courses

Brilliant is an online learning platform that utilizes interactive learning. If you are bored with “traditional” video courses, Brilliant is an exceptional alternative that you may want to consider.

Course Format

All courses on the Brilliant platform will replace video lectures with interactive lessons and assignments. Each of which is thought-provoking. You will then grasp key concepts intuitively.

Below is what you can expect from a Brilliant course.

Each lesson will start with a narrative regarding a real-life scenario, such as a football match. This part will serve as an introduction to explain a particular key concept.

As you continue through that narration, a thought-provoking question will pop up as below. You then need to answer this question. The question is based on the scenario, thus straightforward to answer.

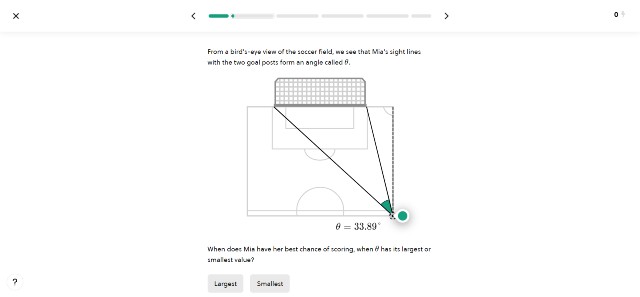
Subsequently, detailed explanations will appear. Based on my observation, they are effortless to understand. You will then start to grasp critical concepts gradually.

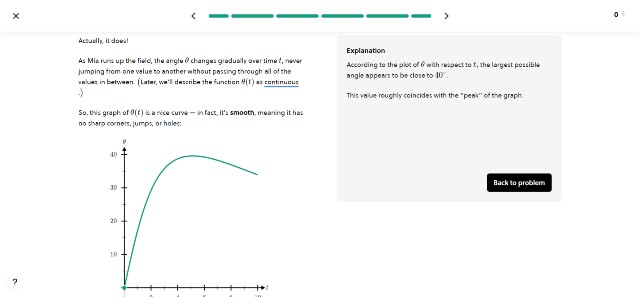
The platform is interactive. For instance, you can freely scroll the bar left or right to modify the charts. These visuals will make the abstract concrete, which aids your understanding.

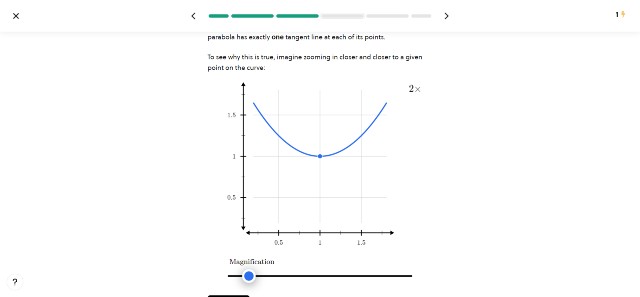
That’s it. This is how Brilliant teaches students. In terms of course structure, each lesson first introduces a real-world case study and then relevant key concepts. This approach is the opposite of traditional courses that always begin with the theoretical.

I find this approach compelling. It can help students understand calculus concepts and applications intuitively.

However, Brilliant has a few drawbacks. The most important is that the platform does not provide many practice problems. Most of which are also too elementary.

Hence, learning from Brilliant will not be sufficient for tough calculus exams like AP Calculus AB/BC or college-level exams.

Course Content

Brilliant offers several calculus courses on its platform, including the following:

Calculus in a Nutshell – This course will introduce you to the core calculus concepts, including limits, derivatives, integrals, and infinite sums, from a bird’s eye view.

Calculus Fundamentals – The second course will explore various methods to compute limits and derivatives. Later, you will learn about linear approximation and implicit differentiation.

Integral Calculus – The third course will elucidate integral calculus core principles and visually perceive how to apply them in various areas, such as physics and probability.

Multivariable Calculus – The fourth course will discuss multivariable calculus. You will intuitively understand how to compute derivatives and integrals for functions with more than one independent variable.

Vector Calculus – This course will cover calculus on vector fields. Apart from critical concepts, you will explore how scientists employ vector calculus in real life.

Pricing

Brilliant uses a subscription-based pricing model. There are three options to consider as follows:

• Annual – $9.99 per month
• Monthly – $24.99 per month
• Group of 3+ – $299.88 per year

Each plan will provide access to all 60+ courses on the Brilliant platform, including calculus, differential equations, statistics, physics, chemistry, Python, and many more.

The annual plan is unarguably the best option price-wise (unless you have two other friends who are willing to subscribe together.)

However, the content on the platform is pretty limited. I think the average student can complete all calculus content platform-wide in a few weeks. Thus, you may not even need an annual plan. The monthly plan is adequate if you don’t plan to take other courses besides calculus.

If you are still unsure whether Brilliant courses are suitable for you, I suggest creating a free account to try free lessons.

Pros & Cons

5. Calculus through Data and Modeling Specialization by JHU

These two Coursera specializations by John Hopkins University (JHU) replace the traditional, theory-centric approach with an application-oriented approach.

Like Krista’s series, both programs focus on helping students master computing techniques, so you will be able to apply them to real-world optimization problems.

Note: You may need a graphing calculator or graphing software on your computer for this course.

5.1) Differential Calculus

This specialization consists of four minor courses as follows:

1. Precalculus – This course will first provide an overview of crucial functions, such as exponential, logarithmic, and trigonometric functions. Later, you will learn about vectors in space and equations of lines and planes.

2. Limits & Derivatives – The second course will introduce you to the concepts of limits and continuity and derivatives are related to them.

3. Differentiation Rules – You will learn about differentiation rules in this course. All of which will help you find derivatives without using the standard equation directly.

4. Applying Differentiation – The final course will focus on applications of differentiation. You will find linear approximations and calculate min/max values for single-variable and multivariable functions.

Unlike other courses featured in this article, this program provides you with several projects to complete (one for each minor course). You will use the knowledge you have learned from the lessons to analyze and manipulate real-world data.

For example, you will find an optimal price point by modeling the costs related to a construction project of a hypothetical company.

Thus, you will quickly grasp how to implement calculus principles in real life, which is excellent if you want to pursue a science-based or economic major in college.

JHU suggests spending 2 hours per week on the course, and you will complete the specialization in six months. However, you can complete it much faster.

Auditing is again free. However, you might want to subscribe to the full course (at $49 per month) to access grading and a digital certificate.

5.2) Integral Calculus

This specialization has a very similar curriculum structure to the above one. It comprises four following minor courses, covering the following topics:

• Sequences and Series
• Fundamental Theorem of Calculus
• Definite/Indefinite Integral
• Iterated Integrals
• Double Integrals Over Plane Regions
• Vector Functions
• Integration Applications – Arc Length, Velocity, Acceleration, etc.
• Vector Calculus – Vector Fields, Line Integrals, Green’s Theorem

Suggested Pace: 2 hours per week for four months, Full access costs $39

Pros & Cons

Advanced Calculus Courses

This section explores in-depth courses on multivariable calculus, vector calculus, and beyond. Hence, these are not for beginners. You should have a solid understanding of the single variable calculus before enrolling.

6. Calculus 3 (Multivariable Calculus) Series

Are you looking for a genuinely in-depth course for multivariable calculus? If that’s the case, I don’t think other online calculus courses suit you more than this Udemy series from Hania Uscka-Wehlou, a Ph.D. who spent years as a senior lecturer at a leading Swedish university.

This series consists of two courses. You will need to purchase them separately.

Note: The series is exceptionally lengthy (the video content alone is almost 100 hours long) and packed with tons of excellent examples and additional learning resources. It is thus optimal for students who want to pursue a math major or steadfast learners.

However, if you are not a math major and just want a crash course to review concepts or prepare for the final exam, Krista’s series (#3) may be more suitable.

Note 2: Besides single variable calculus, you need a solid background in linear algebra before taking the course.

6.1) Part I

Below is a summary of what you will learn from the course.

• Analytical Geometry in the Space – Dot/Cross Product, Scalar Triple Product, Straight Lines in the Plane, Planes/Straight Lines in the Space
• Conic Sections – Circle, Ellipse, Parabola, Hyperbola + Quadric Surfaces
• Coordinate Systems
• Vector-valued Calculus
• Arc Length and Parametrisation
• Functions of several variables
• Deep dive into limits and continuity
• Partial Derivatives, Tangent Plane, Normal Line, Gradient, Jacobian
• Chain Rule
• Linear Approximation, Differentiability, Linearization
• Deep dive into directional derivatives
• Jacobian Determinant and The Implicit Function Theorem
• Taylor’s Formula and Quadratic Forms
• Optimization on open/compact domains
• Lagrange multipliers
• and many more

This course is 47.5 hours long, and that is only the first part! It covers numerous topics that all other courses fail to do so.

Reviews: 4.8/5.0, 1190+ students

6.2) Part II

Part II will delve into integrations and vector calculus. The topics included in the course are as follows:

• Riemann Integrals
• Double Integrals, Integration by Inspection, and Fubini’s Theorem
• Inverse/Direct Substitutions, Change of Variables
• Improper Integrals and Mean Value Theorem
• Triple Integrals
• Applications of multiple integrals – Area, Volume, Surface Area, etc.
• Vector Fields, Schwarz’s Theorem, Conservative Vector Fields
• Line Integrals of Functions, Vector Fields, Path Independence
• Surfaces and Surface Integrals
• Flux Integrals
• Gradient, divergence, and curl
• Green’s Theorem, Gauss’ Theorem, Stokes’ Theorem
• and many more

Besides its highly in-depth lessons, the instructor also provides detailed video solutions. Thus, you can learn all the skills to tackle the toughest of multivariable calculus problems. Furthermore, attached to each course are informative study notes that you can use as a reference.

Reviews: 4.8/5.0, 1190+ students

Pros & Cons

7. Vector Calculus for Engineers

This course from the Hong Kong University of Science and Technology is exclusively created to equip existing or future engineers with vector calculus knowledge.

Upon completion, you will be confident in using them in other essential engineering courses such as fluid mechanics and electromagnetism.

Course Content

The following summarizes the topics you will learn in this course.

• Vectors: Dot/Cross Product, Analytic Geometry of Planes and Lines, Scalar/Vector Triple Product, Scalar/Vector Fields, Short introduction to Matrices
• Differentiation: Partial Derivatives, Least Squares Method, Chain Rule, Triple Product Rule, Gradient, Divergence, Curl, etc.
• Integration and Curvilinear Coordinates – Double and Triple Integrals, Polar Coordinates, Change of Variables, Cylindrical/Spherical Coordinates
• Line and Surface Integrals – Line Integral of a scalar/vector field, arc length, Surface integral of a scalar/vector field, Surface area of a sphere
• Fundamental Theorems – Gradient Theorem, Divergence Theorem, Green’s Theorem, Stokes’ Theorem, Maxwell’s equations, etc.

In essence, this course will prepare you with all the vector calculus knowledge required for the advanced engineering curriculum. Therefore, if you are an engineering student, this course may be most suitable for you.

Completing this course requires approximately 28 hours of commitment. Auditing is free, while a full course costs $49 one-time.

Pros & Cons

Coursera Plus

This article features several Coursera courses and specializations. Suppose you are interested in more than one of them. I recommend replacing a course or specialization subscription with Coursera Plus.

Coursera Plus is a premium subscription that grants full access (not just auditing) to more than 5000 courses (or more than 90% of courses on the platform) at only $399 per year or $33.25 per month.

The fees for Coursera Plus are thus cheaper than a single specialization subscription that costs $39-$79 per month.

Since Coursera Plus offers more courses at cheaper pricing, it is a must-buy for knowledge-hungry students.

Free Online Calculus Courses

8. Single Variable Calculus Series by UPenn

This series by the University of Pennsylvania offers excellent beginner-level calculus training. If you don’t need an extensive algebra review or find the teaching pace of the first course too slow, you may want to give this series a try.

Course Content

This series consists of four Coursera courses. You will need to enroll in each separately.

Part 1 Functions – The first course is essentially a precalculus course. You will learn about the functions, the Taylor Series, and Limits & Asymptotics. The best part is that you will learn the logical process behind calculus, which will significantly help you grasp complex concepts once you reach the advanced level.

Part 2 Differentiation – The second course will delve into differentiation. You will start with methods to find derivatives and differentiation rules. Later, you will learn about differentials and operators.

Part 3 Integration – The third course will drill deep into integration. First, you will learn about differential equations and grasp why we need “anti-differentiation.”

The second section of this course will discuss the Fundamental Theorem of Integral calculus and integration techniques.

Part 4 Applications – The fourth course will explain how professionals use calculus knowledge in real life. This includes the area, volume measurement, physical applications, and many more.

This series essentially covers the same topics as the first course. However, the precalculus section of this series is far less extensive (it does not cover algebra), while the overall pace is also faster. Thus, this series may be more suitable for students with a solid mathematical background.

Unlike other regular Coursera courses and specializations, you can access the entire course (not just audit) for free.

Related Courses

• Linear Algebra
• Differential Equations – Coming Soon